Hi everyone and welcome to MathSux! In today’s post we are going to explore the rules for multiplying radicals, mainly focusing on multiplying binomials expressions that contain radicals. We will go over several types of examples in this post starting with the basics and working our way up to expanding radical binomial expressions and simplifying. If you need a quick review on how to expand binomials using the distributive property/box method, check out this post here. Also, be sure to check out the video and practice questions below. Thanks so much for stopping by and happy calculating! 🙂
What Are the Rules for Multiplying Radicals?
- Multiply the coefficients.
- Multiply the term or terms under the radical.
- Simplify the solution whenever possible.
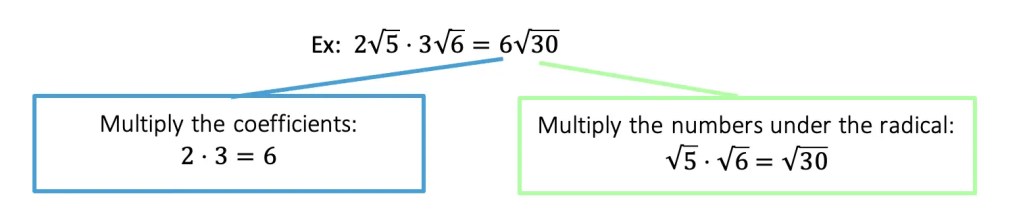
Check out more completed Examples of multiplying radicals that involve some simplifying below:
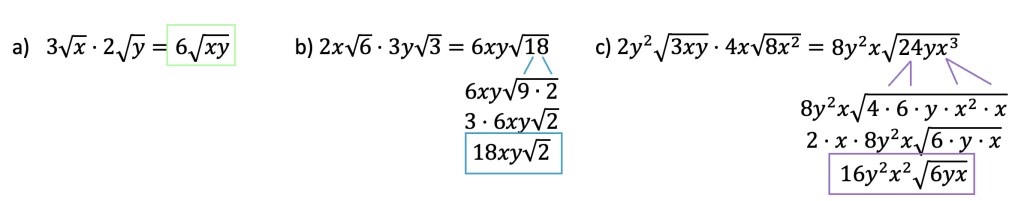
The above shows a few simple cases of multiplying radicals but let us take a look at how to multiply radicals that are placed within a binomial within these next Examples.
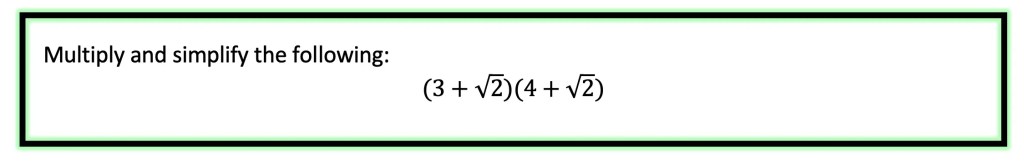
Step 1: To solve this, we are going to use the distributive property to multiply each term by each term, sometimes known as FOIL (for more on the FOIL method, check out this post, here.)

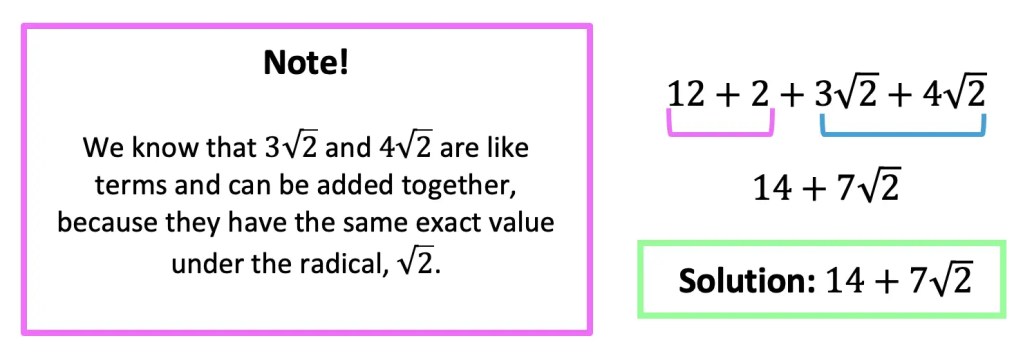
Step 2: Before we say we are done and have a solution, notice that we can simplify this even more by combining like terms, 12+2=14, we can also combine the like radical terms below because they have the same value of 2 under the radical.
Now, let’s take a look at another type of Example, where we need to first expand binomials that contain radicals.
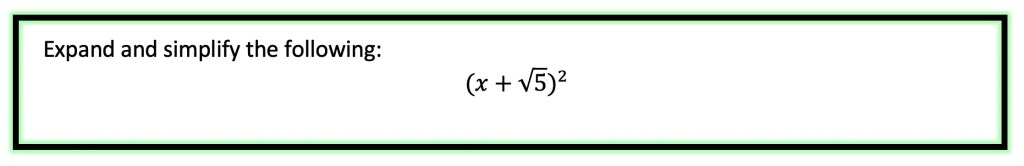
Step 1: Notice in with this example, we are going to need to expand this binomial and then use the distributive property (or FOIL) to multiply each term by each term.
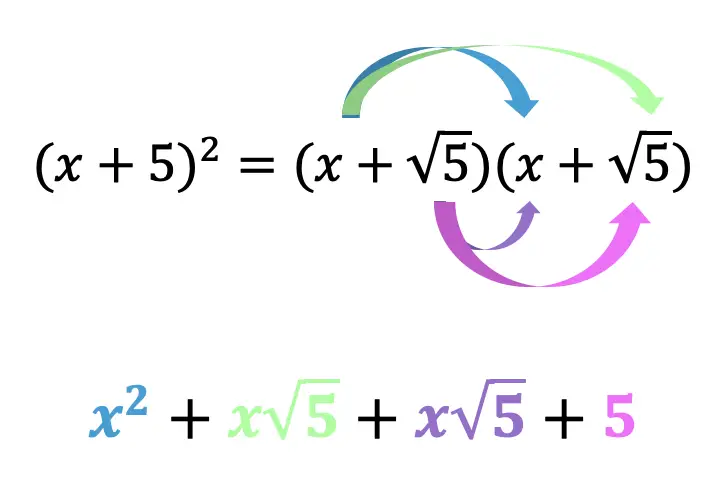
Step 2: In this case, notice that we can simplify our answer even more by combining like terms.
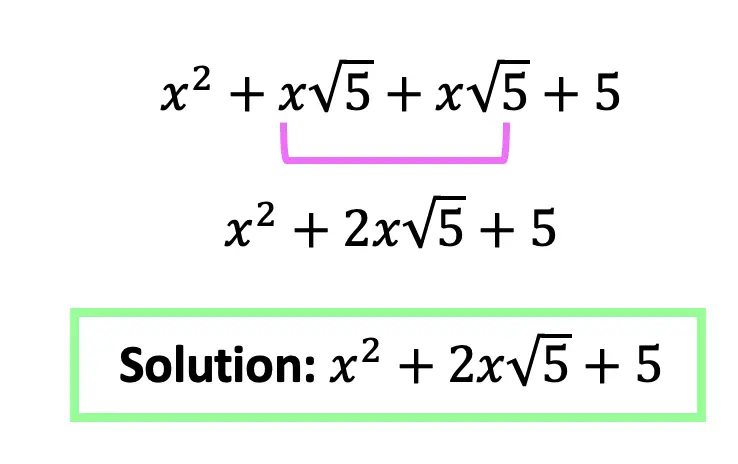
Try the following practice questions on your own to truly master the topic!
Practice Questions:
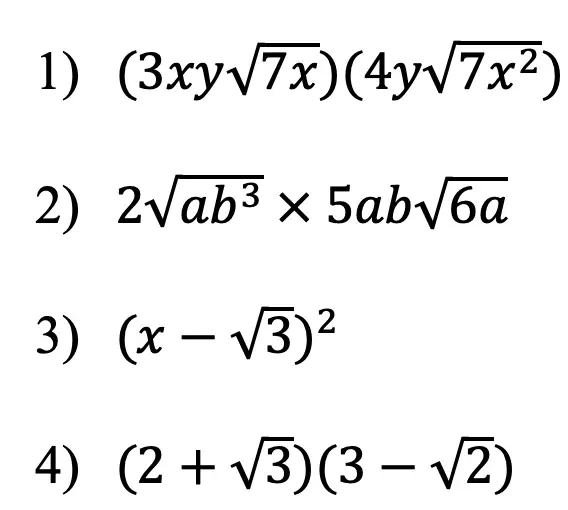
Solutions:

Still got questions? No problem! Don’t hesitate to comment with any questions below or check out the video above. Thanks for stopping by and happy calculating! 🙂

One thought on “Multiplying Radicals Expression”