Happy new year and welcome to Math Sux! In this post we are going to dive right into simultaneous equations and how to solve them three different ways! We will go over how to solve simultaneous equations using the (1) Substitution Method (2) Elimination Method and (3) Graphing Method. Each and every method leading us to the same exact answer! At the end of this post don’t forget to try the practice questions choosing the method that best works for you! Happy calculating! 🙂
What are Simultaneous Equations?
Simultaneous Equations are when two equations are graphed on a coordinate plane and they intersect at, at least one point. The coordinate point of intersection for both equation is the answer we are trying to find when solving for simultaneous equations. There are three different methods for finding this answer:

We’re going to go over each method for solving simultaneous equations step by step with the example below:

Method #1: Substitution
The idea behind Substitution, is to solve for 1 variable first algebraically, and the plug this value back into the other equation solving for one variable. Then solving for the remaining variable. If this sounds confusing, don’t worry! We’re going to do this step by step:
Step 1: Let’s choose the first equation and move our terms around to solve for y.

Step 3: The equation is set up and ready to solve for x!
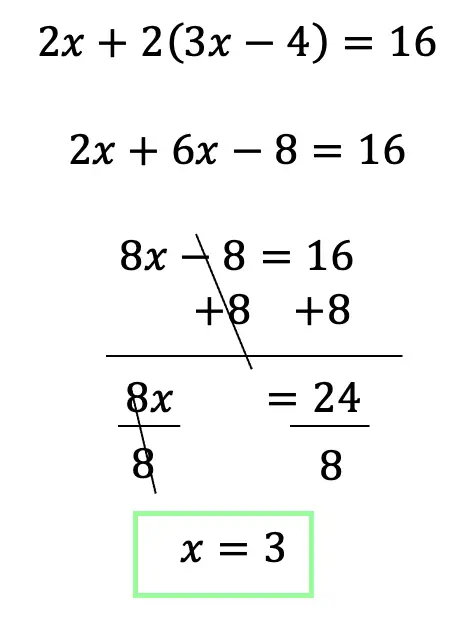
Step 4: All we need to do now, is plug x=3 into one of our original equations to solve for y.
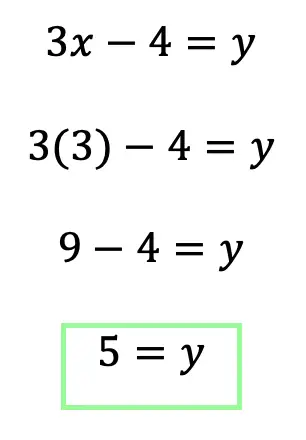
Step 5: Now that we have solved for both x and y, we have officially found where these two simultaneous equations meet!
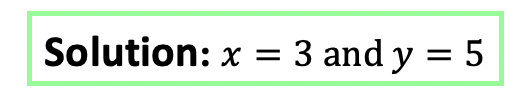
Method #2: Elimination
The main idea of Elimination is to add our two equations together to cancel out one of the variables, allowing us to solve for the remaining variable. We do this by lining up both equations one on top of the other and adding them together. If variables at first do not easily cancel out, we then multiply one of the equations by a number so it can. Check out how it’s done step by step below!
Step 1: First, let’s stack both equations one on top of the other to see if we can cancel anything out:

Step 2: Our goal is to get a 2 in front of y in the first equation, so we are going to multiply the entire first equation by 2.

Step 3: Now that we multiplied the entire first equation by 2, we can line up our two equations again, adding them together, this time canceling out the variable y to solve for x.
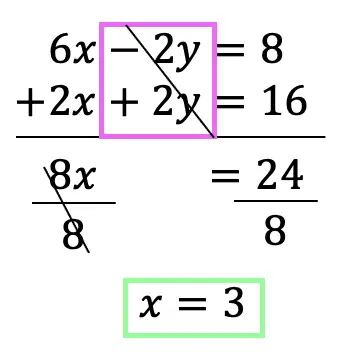
Step 4: Now, that we’ve found the value of variable x=3, we can plug this into one of our equations and solve for missing unknown variable y.
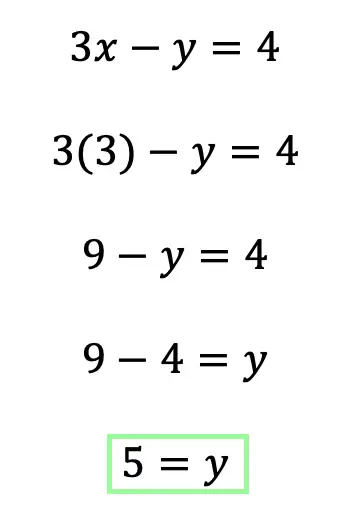
Step 5: Now that we have solved for both x and y, we have officially found where these two simultaneous equations meet!
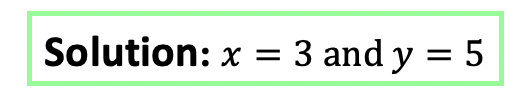
Method #3: Graphing
The main idea of Graphing is to graph each a equation on a coordinate plane and then see at what point they intersect. This is the best method to visualize and check our answer!
Step 1: Before we start graphing let’s convert each equation into y=mx+b (equation of a line) form.
Equation 1:

Equation 2:
Step 2: Now, let’s graph each line, y=3x-4 and y=-x+8, to see at what coordinate point they intersect.
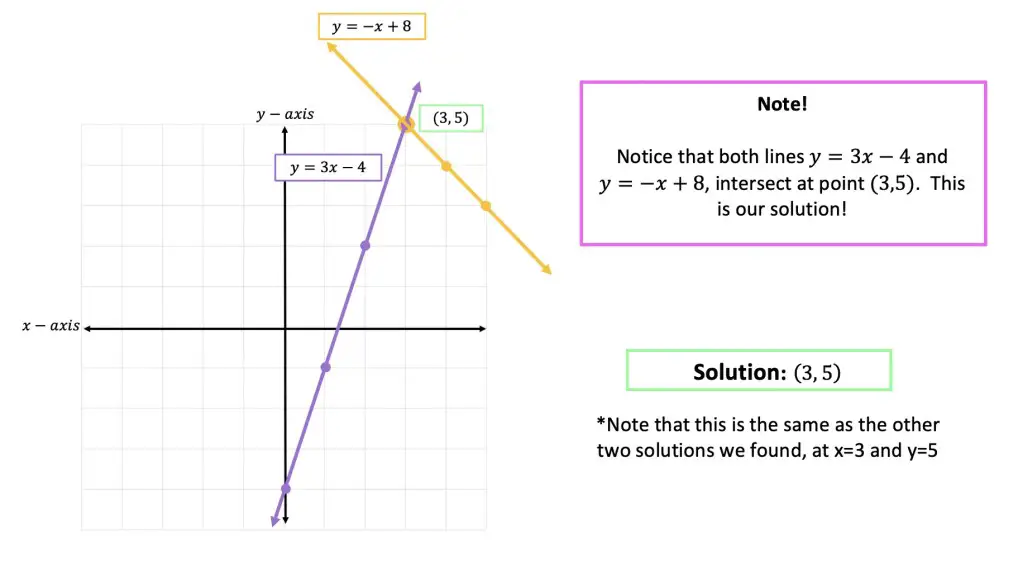
Need to review how to draw an equation of a line? Check out this post here! Notice we got the same exact answer using all three methods (1) Substitution (2) Elimination and (3) Graphing.
Ready to try the practice problems on your own?! Check them out below!
Practice Questions:
Solve the following simultaneous equations for x and y.
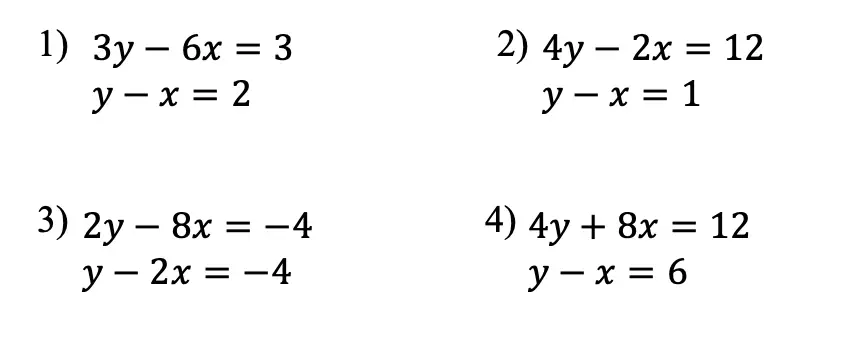
Solutions:
- (1, 3)
- (4,5)
- (-1, -6)
- (3, -3)
Want more MathSux? Don’t forget to check out our Youtube channel and more below! And if you have any questions, please don’t hesitate to comment below. Happy Calculating!
Facebook ~ Twitter ~ TikTok ~ Youtube
If you are looking for a challenge, try solving three equations with three unknown variables, in this post here!

One thought on “Simultaneous Equations: Algebra”