Trig Identities

Hi everyone and welcome to MathSux! In today’s post, we are going to dip our toes into Trig Identities! There are a ton of trig identities out there but six trig functions that you’ll need to know for trig identity proofs, luckily most of them are related to the trigonometric functions you are probably already familiar with! Trig proofs involve working with trig functions we are already familiar with (sin θ, cos θ, tan θ), but breaking them down, inverting each trig function, and applying rules that are always true (otherwise known as trigonometric identities).

Proofs can feel a bit tricky at first, but with some practice they should start to feel more normal! The key is to always leave one side of the equation alone, while working with and manipulating the other side of the equation until it matches the other.

This is a great topic for getting more familiar with trig without actually having to work with any triangles. If you are familiar with the trig identities below you should feel good about answering these types of questions. Below you’ll also find a trig identity cheat sheet and a list of even more trigonometric identities that you may need! Also, don’t forget to check out the video and practice questions below to master trigonometric identities. Happy calculating!

Trig Functions Identity Cheat Sheet:

Trig-Identity-Cheat-Sheet-3 Download

Inverse Trigonometric Identities:

Inverse Trigonometric Identities, are the inverses of the same trig functions we all know and love (sin θ, cos θ, tan θ). When we take the inverse of sin θ, cos θ, tan θ, we end up getting these new trig functions: csc θ=1/sin θ, sec θ=1/cos θ, and cot θ=1/tan θ! The trig functions and their inverses should all look familiar, so memorizing them should piece a piece of cake! Simple? Yes! These are a big key to solving many trig identity proofs.

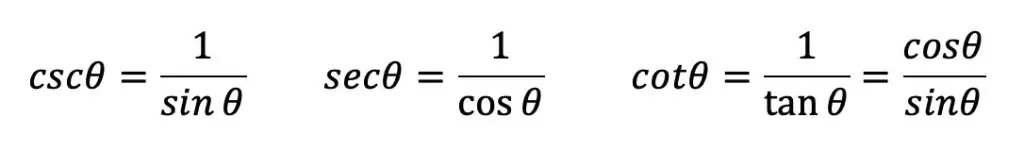
Trigonometric Ratio Identities:

Trigonometric Ratio Identities are great for breaking down tan θ and cot θ into tan θ=sin θ/cos θ and cot θ=cos θ/sin θ. Notice that tan and cot are the reciprocal of one another, all we need to do is remember the following:

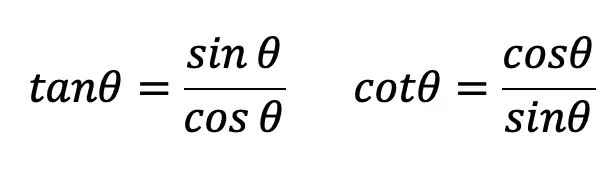
Trigonometric Pythagorean Identities:

Trigonometric Pythagorean Identities are based on none other than the pythagorean theorem in relation to trigonometry and the unit circle. The main equation to know for all of the listed Pythagorean Identities, is sin² θ+cos² θ=1. Knowing this one equation allows us to derive 8 more related pythagorean identity functions which will ultimately help us when breaking down trig functions in our trigonometric identity proofs. Below front and bolded is our main Pythagorean Identity:

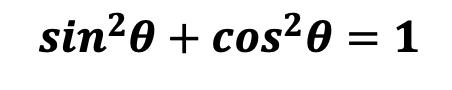
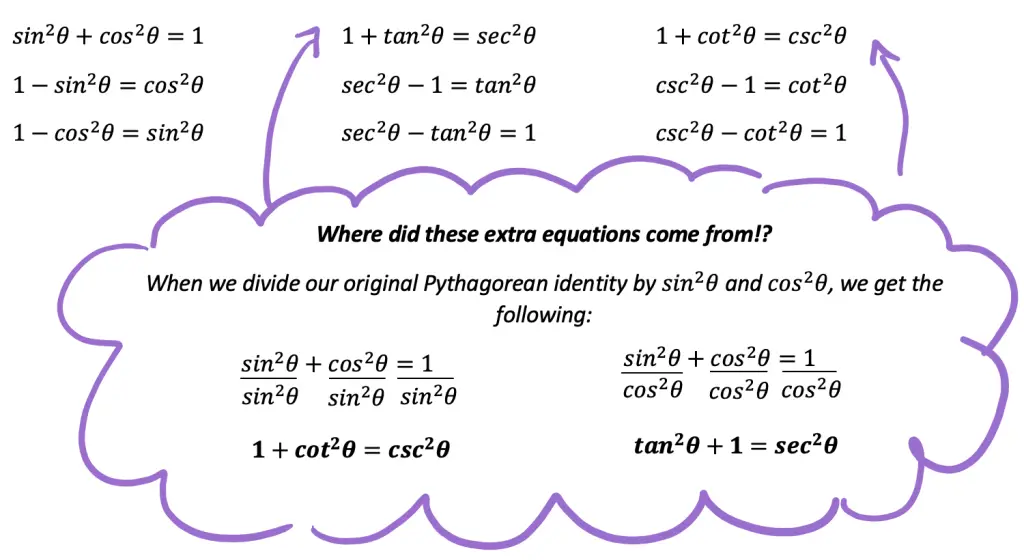
Now that we have everything we need to prove trig identities true, let’s apply our new knowledge and take a look at an example below:

Example:

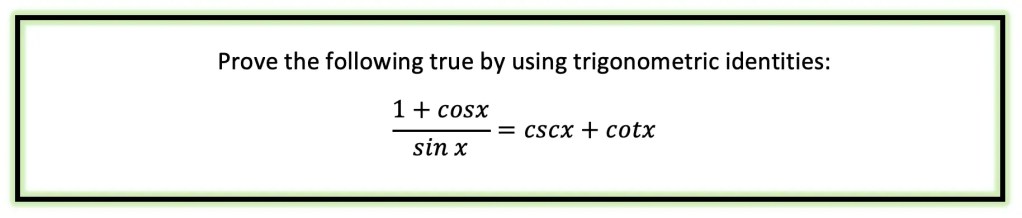
Step 1: First, we are going to focus on the left side of the equation only, trying to get it equal to the right side, leaving csc θ+cot θ unchanged.

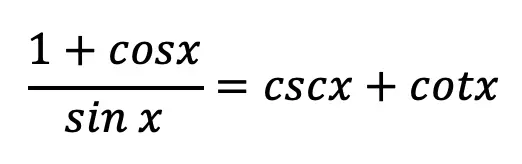
Step 2: Notice, we can expand the left side of the equation out, separating the sinθ in the denominator under each term of the numerator. This is based on basic rules for adding and subtracting fractions…nothing new or related to trig functions yet!

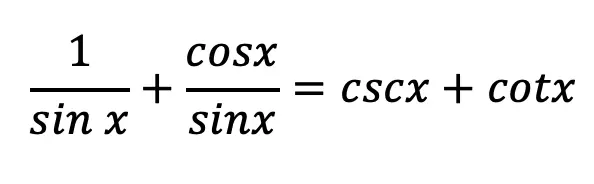
Step 3: Now, looking at our trigonometric inverse and ratio identities, we can see that 1/sin θ can be re-written as the trig functions inverse identity, csc θ and that cos θ/sin θ can be re-written as trigonometric ratios identity as cot θ. Let’s re-write those and fill them in below to get the correct answer and prove our trigonometric functions equation true:

Ready for more examples to try on your own!? Check out the practice problems below:

Practice Problems:

Prove each of the following true, then check your answer, with each worked through trig functions identity proof below.

Solutions:

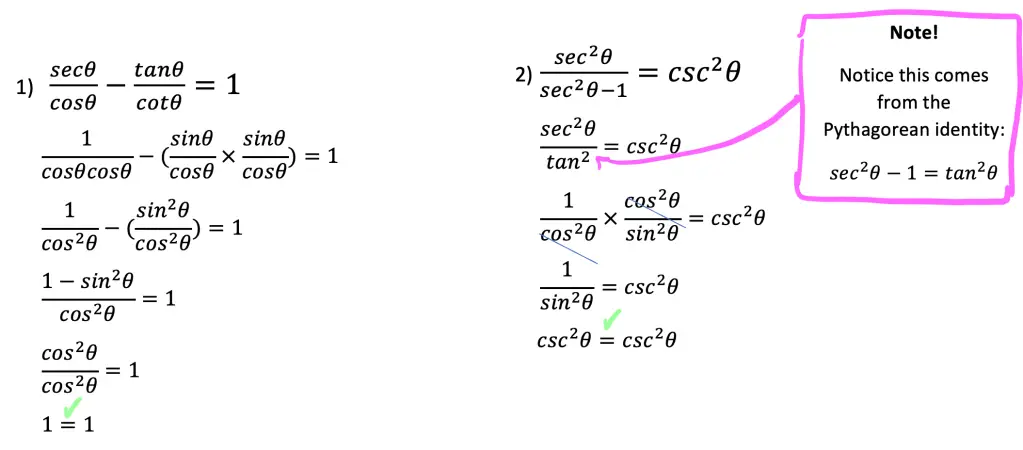
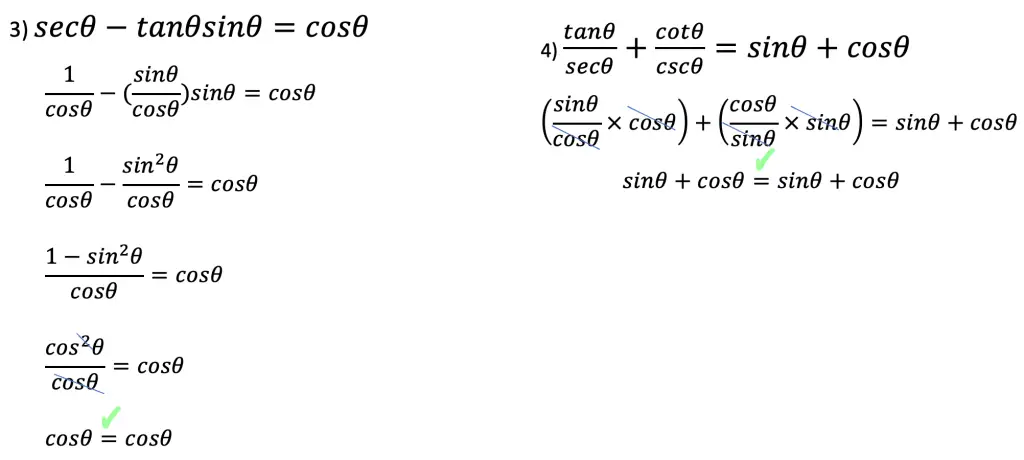
Looking for more trigonometric identities? Check these out below for your reference! The trigonometric identities below are used for specific questions separate from the proof examples shown earlier in this post. But there will come a time when these will be needed so hold on to them for now and let me know if you’d like to see some examples!

Co-Function Identities:

Each of the Co-Function Identities below represents each basic trig function and their corresponding complementary angle, meaning that they each add to 90º (or in radians π/2).

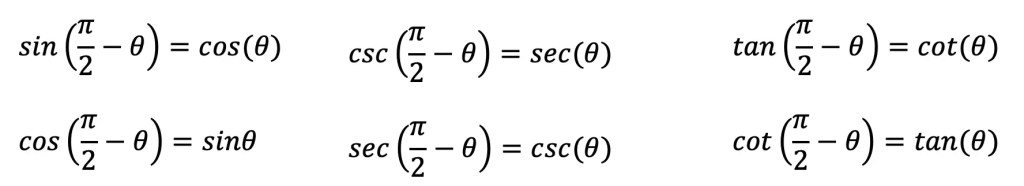
Half Angle Identities:

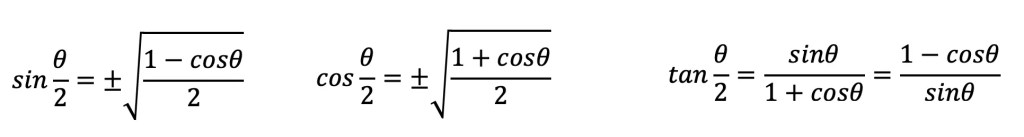
Double Angle Identities:

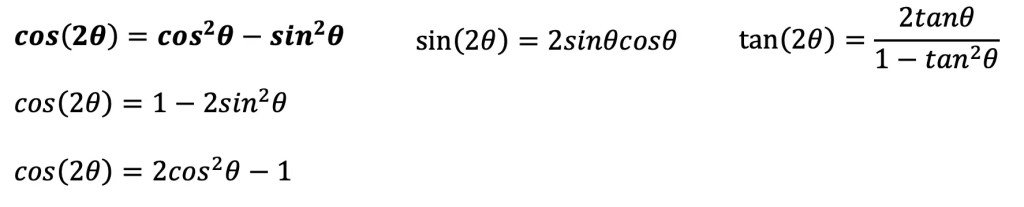
Sum and Difference Identities:

Also known as ptolemy’s identities, the following are the sum and difference formulas for sine and cosine.

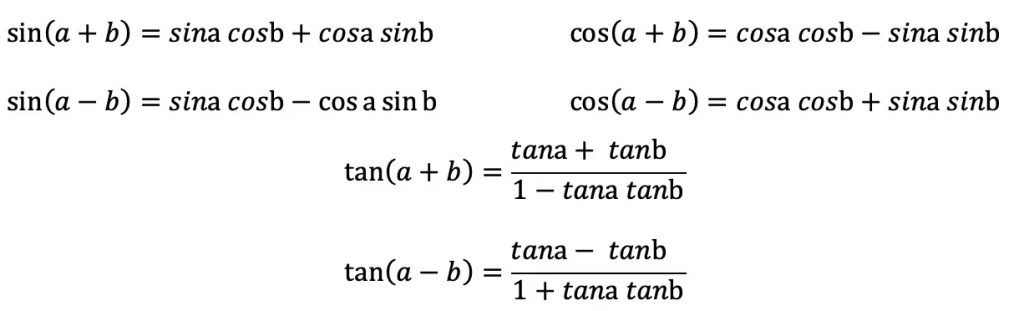
Still got questions? Have a favorite trigonometric identity? Don’t hesitate to email or comment below with any questions to clear things up! Happy calculating!

Also, don’t forget to follow us on social media to get the latest and greatest MathSux lessons, videos, questions, and more!

Facebook ~ Twitter ~ TikTok ~ Youtube