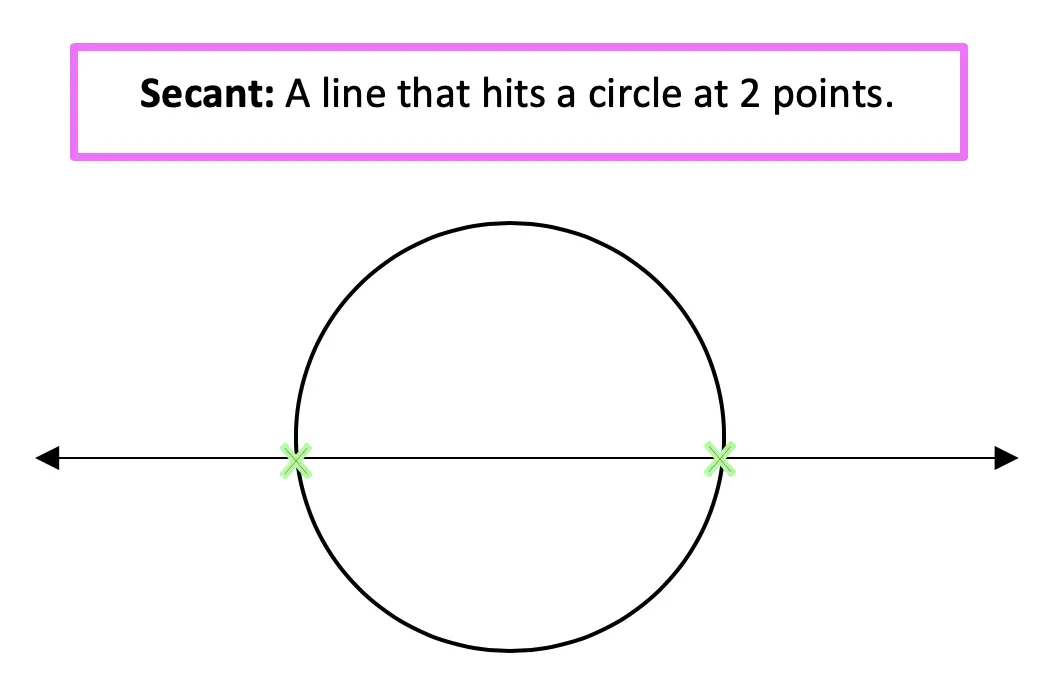
Ahoy! Today we’re going to cover the Intersecting Secants Theorem! If you forgot what a secant is in the first place, don’t worry because all it is a line that goes through a circle. Not so scary right? I was never scared of lines that go through circles before, no reason to start now.
If you have any questions about anything here, don’t hesitate to comment below and check out my video for more of an explanation. Stay positive math peeps and happy calculating! 🙂
Wait, what are Secants?
Intersecting Secants Theorem: When secants intersect an amazing thing happens! Their line segments are in proportion, meaning we can use something called the Intersecting Secants Theorem to find missing line segments. Check it out below:

Let’s now see how we can apply the intersecting Secants Theorem to find missing length.
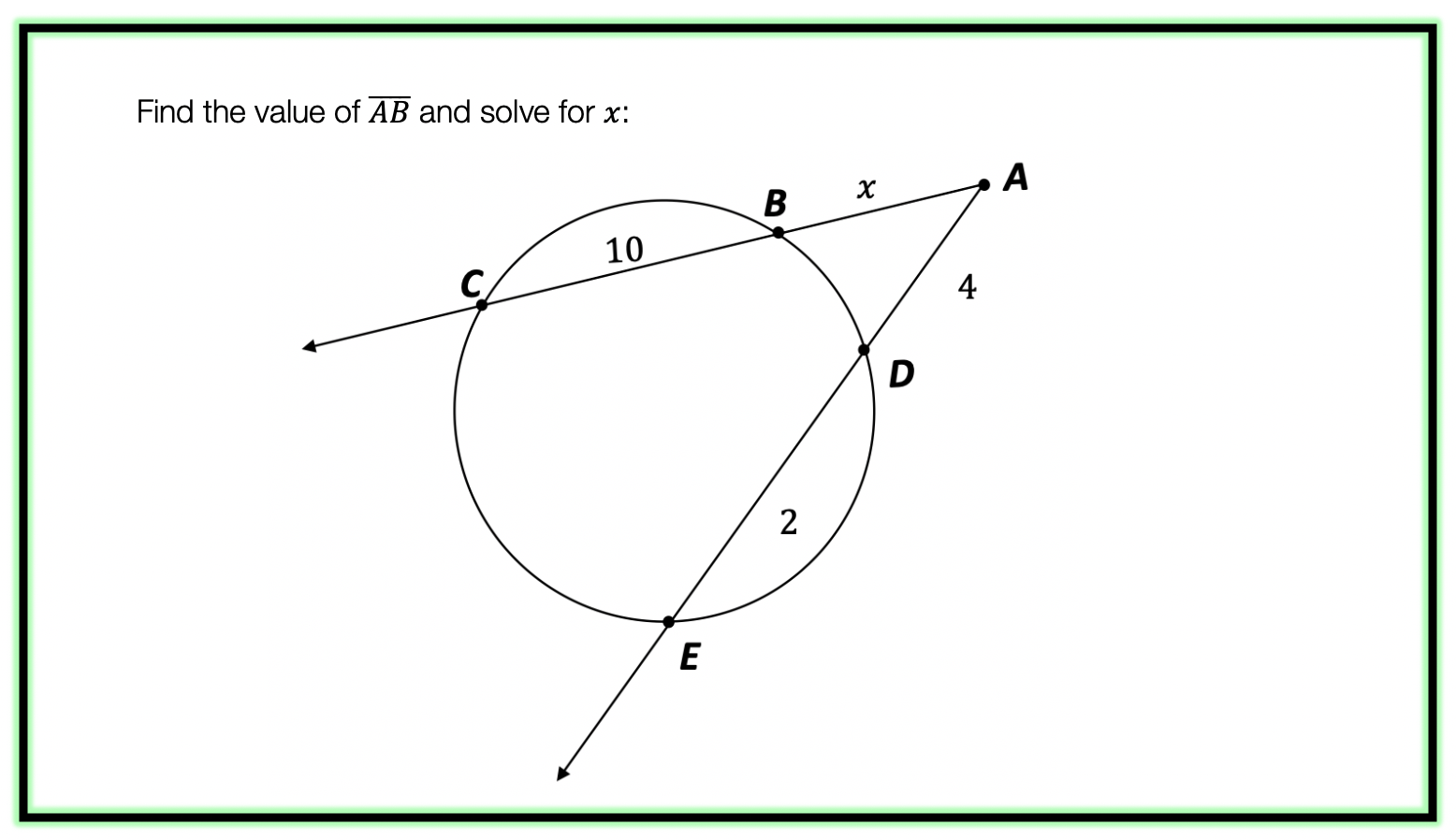
Step 1: First, let’s write our formula for Intersecting Secants.
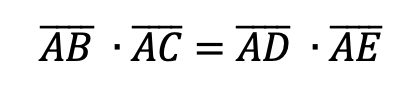
Step 2: Now fill in our formulas with the given values and simplify.
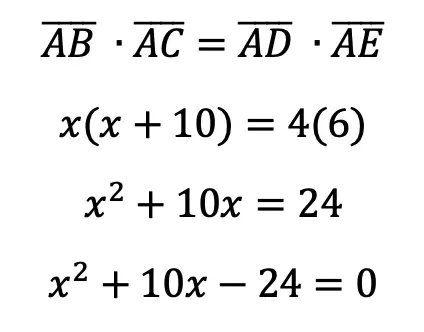
Step 3: All we have to do now is solve for x! I use the product.sum method here, but choose the factoring method that best works for you!
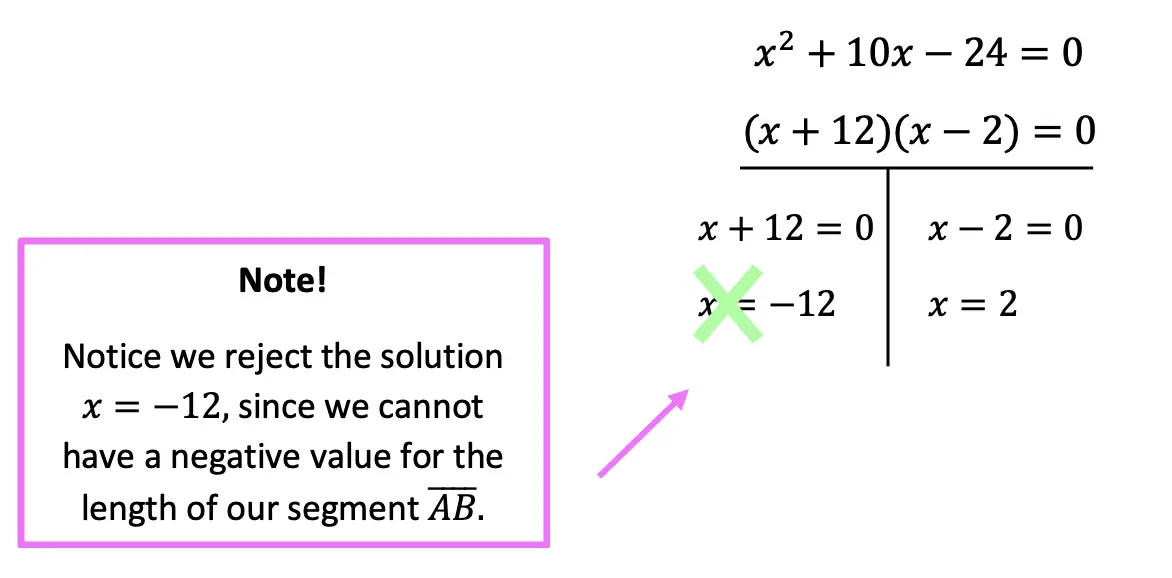
Step 4: Since we have to reject one of our answers, that leaves us with our one and only solution x=2.

Ready to try the practice problems below on your own!?
Practice Questions: Find the value of the missing line segments x.
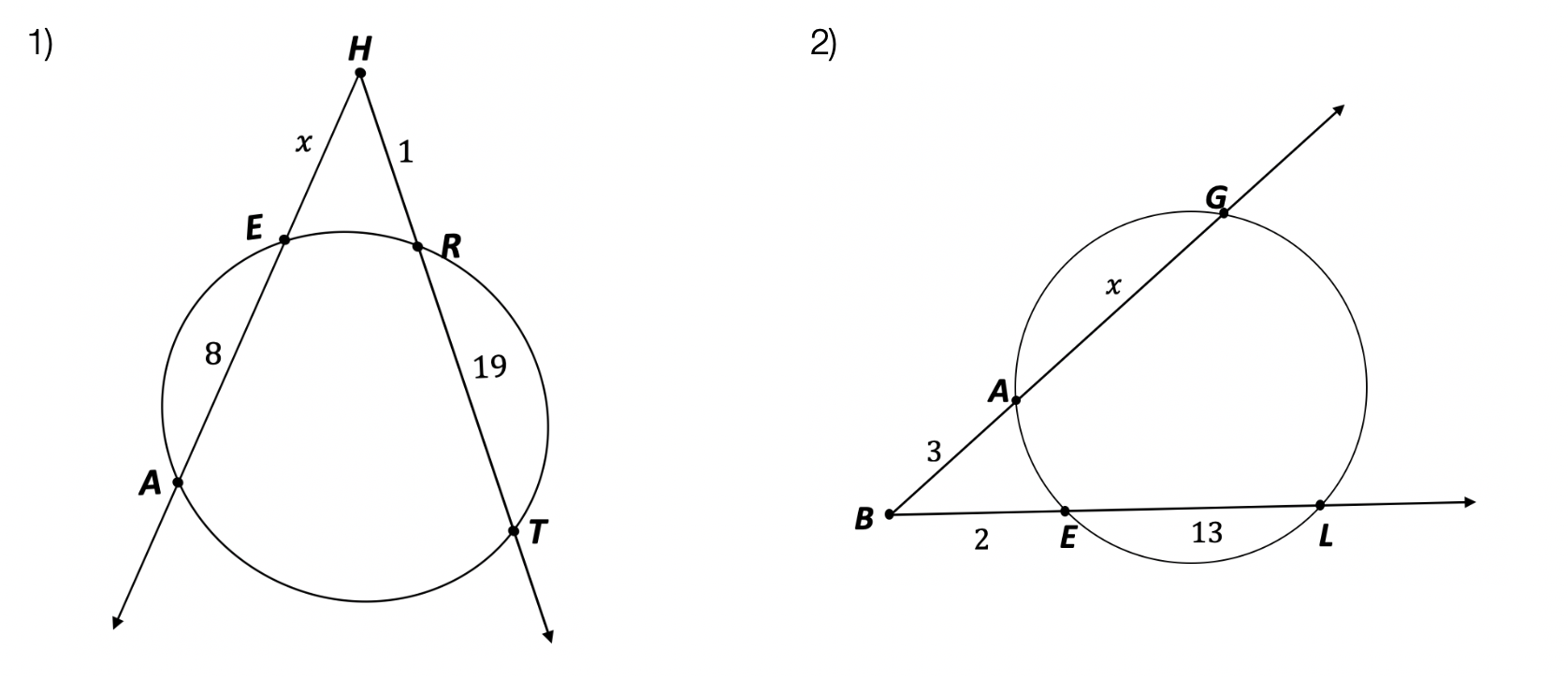
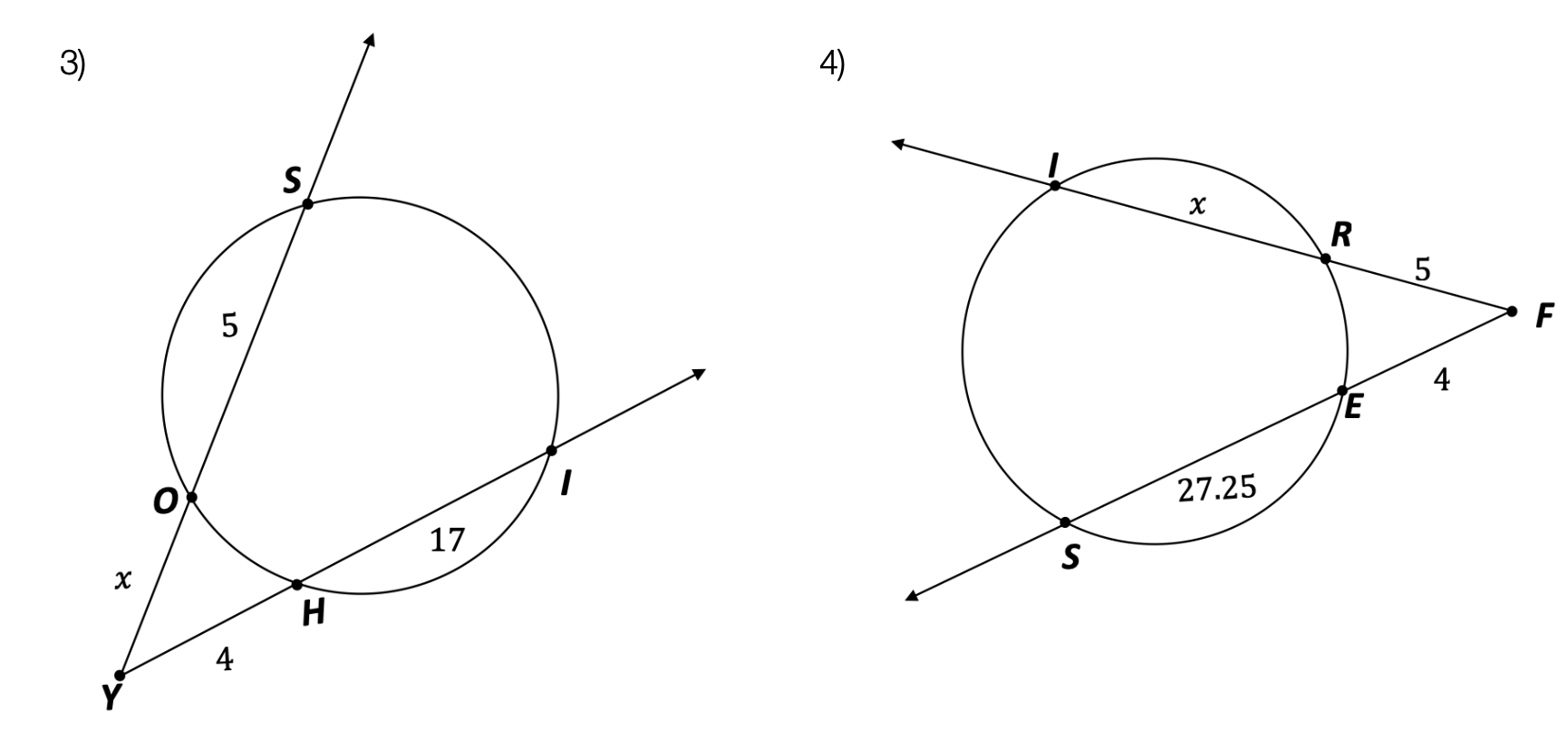
Solutions:
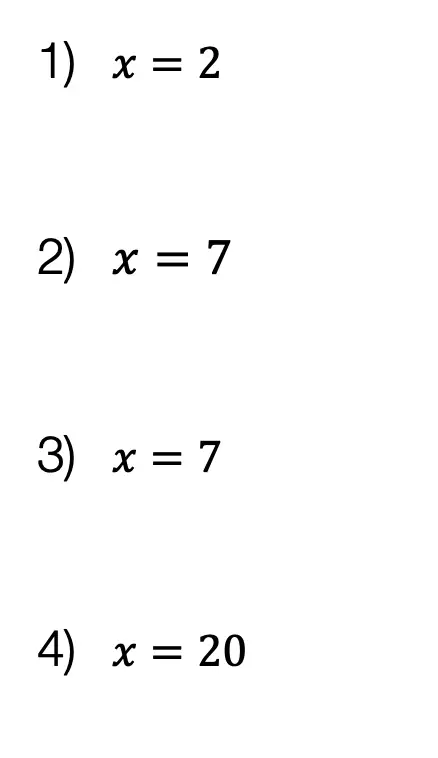
Still got questions? No problem! Check out the video above or comment below for any questions and follow for the latest MathSux posts. Happy calculating! 🙂
Facebook ~ Twitter ~ TikTok ~ Youtube
To review a similar NYS Regents question check out this post here.

3 thoughts on “Intersecting Secants Theorem: Geometry”